Post by: cheryl j on 01/02/2012 20:09:25
It was only when I took chemistry and physics in highschool that I developed any appreciation for or enjoyment of maths at all. And really, I only liked physics and chemistry because it helped me understand biology better, which is what I really loved.
But to this day, maths haunts me, because I feel like I missed out on some other way of understanding or experiencing the world, like a deaf person who can't hear music. Especially when maths people talk about the beauty or "elegance" of an equation, and I have no idea what they mean. The other thing I don't understand is when maths people talk about having an instinct or a "feel" for how certain mathematical things work. I'm ashamed to admit I took an entire course in calculus and had no idea why it was invented or what the point of it was. I just copied the steps the professor did on the black board, and tried to duplicate it on the test.
I don't think I am a stupid person, but I have to admit, when it comes to maths, I feel like part of my brain never developed at all. The biologist in me would like to know what differences there are in the brains of people who are good at maths and those who are not, and if it can be changed, or its hardwired.
Post by: wolfekeeper on 02/02/2012 06:11:24
The point of maths is (more or less) that you abstract patterns from real world things and try to work on the patterns independently from the the thing you took the pattern from. To the extent that you can do that, you can use the same maths across different many places; places where you wouldn't normally expect there to be any connections.
Numbers aren't actually that much to do with higher mathematics, at the very highest levels it's more like a foreign language, so it may even be that you would be bad at the lowest levels, but good at the higher levels.
The other thing is, that's nobody really, really good at maths, since mathematicians have managed to prove that maths is infinitely hard; so there's always an equation so hard that no mathematician will ever prove it.
Post by: CliffordK on 02/02/2012 08:28:49
For example, setting the volume of a Black Hole to zero doesn't mean that it in fact exists.
And, while I used to like mathematics, I do have a bad habit of jumping to the answer and ignoring the derivation.
Everything depends on what you wish to do, and are comfortable with. I do find that algebra and trigonometry is much more useful for my current needs than calculus. And, I probably learned more about Algebra in Calculus than I did in Algebra. However, it doesn't hurt to at least understand the basic slopes, areas, and state changes that one derives with calculus.
If I need to solve a series of linear equations, I'm much more likely to use brute force than complex matrix transformations.
A year or so ago I thought I'd try some science fiction writing.
While I'm not convinced that the speed of light is an absolute limit, it quickly became obvious how difficult of a task it is to get anywhere near the speed of light. And, my aspirations to become a science fiction writer soon became Epsilon!!!! So much for Math actually helping a person [xx(]
Post by: krool1969 on 02/02/2012 08:44:42
Post by: graham.d on 02/02/2012 10:00:43
Post by: wolfekeeper on 02/02/2012 21:20:00
Especially when math people talk about the beauty or "elegance" of an equation, and I have no idea what they mean.Usually they just mean it's a short equation(!) Often the symbols take several pages to understand though, so the elegance is sometimes illusory. And you shouldn't assume that the mathematicians are consistent about it, and I've seen equations that are shorter and more useful that many mathematicians didn't like, for historical reasons only.
Post by: Geezer on 02/02/2012 21:38:41
I'm ashamed to admit I took an entire course in calculus and had no idea why it was invented or what the point of it was.
I don't think you are alone! We did some calculus in high school, but I didn't see the point in it until later when I got into engineering. When I understood how it could be applied to solve real problems, the light bulb went on. I suspect our teacher didn't have much sense of how it could be applied either.
Post by: David Cooper on 02/02/2012 22:11:58
Post by: wolfekeeper on 02/02/2012 22:34:37
Well, not alien, maths is a foreign language, and if you lose the sense of what it means, you'll not be able to do good maths. But you should always remember that maths is about patterns, not about the thing you're trying to do maths on, and the patterns have a life of their own.
Post by: wolfekeeper on 02/02/2012 22:40:58
Newton got hung up on the examples he used to derive it; calculus was invented to deal with the motion of planets and things, so everything was about rates of change with respect to time.
Leibnitz was more general, he allowed rates of change with respect to anything, like 'how does gravity change with the distance away from a planet', but Newton couldn't get his head around those kinds of rates of change, only the rates with respect to time; his examples always were speeds (rate of change of distance with time) and accelerations (rate of change of speed with time). He called them 'fluxions'.
So the calculus we use comes from Leibnitz.
Post by: David Cooper on 02/02/2012 23:05:19
The main point of maths is to turn it into alien squiggles.
It's fine to use alien squiggles after the maths that lies behind them has been explained to the point that you have a proper understanding of it, but many teachers go straight into dx/dy which doesn't appear to have anything to do with dividing the upper by the lower term, then change the d into greek for some reason, and then they start hurling barless "f"s around and talking about limits without ever demonstrating that any of it can do anything useful. Before long, the teacher takes to writing something complex on the board and asking you to simplify it. When she finally demonstrates how it should be done, she generates something twice as complex as the thing she was claiming to simplify, and none of it appears to make any sense because she hasn't explained it. Everyone else in the class either gets their parents to pay for tutoring or they just give up.
Quote
Well, not alien, maths is a foreign language, and if you lose the sense of what it means, you'll not be able to do good maths.
It wasn't losing the sense of it that was the problem - it was trying to get the sense of it in the first place. My "teacher" didn't give me anything to lose.
Quote
But you should always remember that maths is about patterns, not about the thing you're trying to do maths on, and the patterns have a life of their own.
The people who worked out calculus did it to solve specific problems and they knew exactly what those problems were. Why shouldn't everyone else be allowed to start off at the same point where there are clear problems there to be solved and where they can see a mathematical process generate answers. It should be possible to program a computer to solve these problems without having to type alien squiggles in at all - all of it must be possible using nothing more than a standard CPU instruction set.
Post by: wolfekeeper on 03/02/2012 00:38:01
But it's not just divide one by the other, it's defined as the limit of dividing dy/dx as you take dx towards zero.
Limits are answers where you find that the answer gets closer and closer to something, without ever quite getting there, (if it actually got there the maths usually explodes, but that doesn't matter!!) But not every equation tends to a limit, some don't converge to a limit at all, and some give you a different answer depending on which side of the limit you start from.
For example:
y = (x+1)/x
y tends to 1/x as x tends to 0, but when you get to 0, it explodes! If you don't believe me, try plugging in a small x and seeing what y is, you'll find it's very close, and gets closer the smaller x is. The reason is, is because when x is small x+1 in the numerator is basically 1, but x in the denominator is still significant to the answer.
That's what calculus is all about, it's based on limits. dy/dx is short hand for saying take dx towards zero and dy with it, what do you get when you divide them.. in the limit?
So for example in your gravity simulation dx/dt is the speed of a body; if you set dt very small, how fast is the body moving? dx/dt! It's just distance (dx) over time (dt), speed!
It turns out, like with the (1+x)/x case above, with calculus you can solve some important equations exactly using limits, but don't worry, many equations are still impossible to solve!
Post by: Geezer on 03/02/2012 07:32:04
That's what calculus is all about, it's based on limits. dy/dx is short hand for saying take dx towards zero and dy with it, what do you get when you divide them.. in the limit?
There you go. That is not what calculus is "all about".
dy/dx is simply a method of determining the slope of something at a particular place on the slope. The "limits" are simply an artifact of the method employed.
I'm not suggesting that "limits" are not required, but the whole point of calculus was to solve practical problems. Limits fell out of that, not the other way around.
This is precisely why so many people become disenchanted when mathematicians ennoble the method rather than the purpose.
Post by: wolfekeeper on 03/02/2012 07:51:53
I don't think it's possible to do calculus without limits, and having some understanding of them; I think everyone that has ever done calculus gets shown a right-angle triangle of sides dy and dx and is asked to imagine what happens as you make that triangle ever-so-small. That's just exactly the same as taking the limit.
Post by: Geezer on 03/02/2012 08:12:12
Well, if as you say and I say, limits are required to do the job, then they're required to do the job.
I don't think it's possible to do calculus without limits, and having some understanding of them; I think everyone that has ever done calculus gets shown a right-angle triangle of sides dy and dx and is asked to imagine what happens as you make that triangle ever-so-small. That's just exactly the same as taking the limit.
Of course it is, but the entire purpose of the exercise is to determine the slope (the rate of change of something with respect to something else) during a very small change in either one of them. That's why the differentiation was invented.
Ironically, Newton didn't think it was any big deal. He just cooked it up as a method to help him solve some problems.
Post by: wolfekeeper on 03/02/2012 08:30:55
The people who worked out calculus did it to solve specific problems and they knew exactly what those problems were. Why shouldn't everyone else be allowed to start off at the same point where there are clear problems there to be solved and where they can see a mathematical process generate answers.Yes, most people learn general processes by starting with the specific and learning how to generalise them.
Quote
It should be possible to program a computer to solve these problems without having to type alien squiggles in at all - all of it must be possible using nothing more than a standard CPU instruction set.Both yes, and no. Mainly no.
Normal calculus takes an equation, and changes the equation 'differentiating' or 'integrating' to give new equations. Computers can do that, but those are very definitely alien squiggles it's manipulating, you type them in in that form, and you get them back in that form.
The other way to do it is not to use true calculus, but to use small deltas. So you divide the problem up into small chunks, but you do not take it down to the infinitesimal limit. If the distances between the chunks are small enough, this approximates to using calculus. That's done rather a lot, it's called 'finite element analysis' and similar names.
Post by: Geezer on 03/02/2012 08:48:27
Computers can do that, but those are very definitely alien squiggles it's manipulating, you type them in in that form, and you get them back in that form.
Only because the computer translates them to and from mathematical hieroglyphs in the process. The computer is only capable of operating on a series of very mundane mathematical expressions.
Analog computers did implement calculus in hardware, but I don't think any of the digital variety do that.
Post by: wolfekeeper on 03/02/2012 17:44:52
Analogue computers and finite element analysis can both do very much the same thing, except that one is sampled and the other is continuous, but that doesn't matter provided the Nyquist criteria is satisfied.
Post by: David Cooper on 03/02/2012 22:06:30
Limits are answers where you find that the answer gets closer and closer to something, without ever quite getting there, (if it actually got there the maths usually explodes, but that doesn't matter!!) But not every equation tends to a limit, some don't converge to a limit at all, and some give you a different answer depending on which side of the limit you start from.
I thought the whole purpose of calculus was the it could get to an exact answer at some point rather than just getting a good approximation. For example, if you're trying to calculate the slope of a curve through a point, you can calculate the slope of a line and make that line shorter and shorter to get a closer and closer approximation of the slope at the point, but you can't do anything useful with a line of zero length. I was led to believe that calculus would somehow enable you to get an exact value for the slope at a point, but I never saw any evidence that this was actually possible during hundreds of hours of watching a teacher fail to explain how calculus was meant to be done.
Quote
For example:
y = (x+1)/x
y tends to 1/x as x tends to 0, but when you get to 0, it explodes! If you don't believe me, try plugging in a small x and seeing what y is, you'll find it's very close, and gets closer the smaller x is. The reason is, is because when x is small x+1 in the numerator is basically 1, but x in the denominator is still significant to the answer.
I can see what happens with different numbers in that, but I can't relate it to anything in the real world, and that's a large part of my problem - I can handle the abstract once I've seen it being applied to something real, but so long as it is nothing but abstract it is impossible for me to tie it to anything in my head.
Quote
So for example in your gravity simulation dx/dt is the speed of a body; if you set dt very small, how fast is the body moving? dx/dt! It's just distance (dx) over time (dt), speed!
It would be helpful if this stuff was taught by retaining words in the early stages so that you can remember what things are meant to be. Why not just write dist/time? And why does it suddenly go Greek with delta replacing d? The trouble I have with calculus is may be mainly down to a teacher introducing symbols and never restating what they're supposed to mean.
It should be possible to program a computer to solve these problems without having to type alien squiggles in at all - all of it must be possible using nothing more than a standard CPU instruction set.Both yes, and no. Mainly no.
Normal calculus takes an equation, and changes the equation 'differentiating' or 'integrating' to give new equations. Computers can do that, but those are very definitely alien squiggles it's manipulating, you type them in in that form, and you get them back in that form.
If it can be done at all, it must be possible for a computer to do the job with a set of very simple instructions. It has to be able to turn the squiggle version of the problem into something it can handle through those simple instructions, and that simply requires someone to program it to decode the squiggles. My problem is that no one has ever told me how to decode the squiggles in a way that's intelligible to anyone who doesn't already know how to do it.
Quote
The other way to do it is not to use true calculus, but to use small deltas. So you divide the problem up into small chunks, but you do not take it down to the infinitesimal limit. If the distances between the chunks are small enough, this approximates to using calculus. That's done rather a lot, it's called 'finite element analysis' and similar names.
If a human can work out exact answers using calculus, why would a machine not solve problems the same way? If a human can do it, it ought to be dead easy for a machine. Maybe it's faster to do it with approximations and that might matter in something like graphics for computer games where precise answers may be unnecessary.
Post by: wolfekeeper on 03/02/2012 22:20:00
Because if you do dist/time, that's actually the average speed. What you're trying to calculate is the speed at a particular instant in time. For example, a car that is accelerating may travel 100 metres in 10 seconds, but it wasn't going at 10m/s the whole way. At the beginning it was stationary, at the end it was going at (say) 20 m/s.QuoteSo for example in your gravity simulation dx/dt is the speed of a body; if you set dt very small, how fast is the body moving? dx/dt! It's just distance (dx) over time (dt), speed!
It would be helpful if this stuff was taught by retaining words in the early stages so that you can remember what things are meant to be. Why not just write dist/time?
How would you work out the speed at the end of the course, if it wasn't accelerating completely uniformly?
Quote
And why does it suddenly go Greek with delta replacing d? The trouble I have with calculus is may be mainly down to a teacher introducing symbols and never restating what they're supposed to mean.Delta means it's not a limit, it's a real number you could type into your calculator, like 0.0001, it's just a small number.
Quote
No, it doesn't decode them, it treats them as squiggles and messes around with the squiggles. It doesn't have a clue what the squiggles really mean.Normal calculus takes an equation, and changes the equation 'differentiating' or 'integrating' to give new equations. Computers can do that, but those are very definitely alien squiggles it's manipulating, you type them in in that form, and you get them back in that form.
If it can be done at all, it must be possible for a computer to do the job with a set of very simple instructions. It has to be able to turn the squiggle version of the problem into something it can handle through those simple instructions, and that simply requires someone to program it to decode the squiggles.
Quote
In most real-world cases humans can't work out exact answers either. Maths only works in certain situations, mostly the ones you get shown in school! Usually in the full glorious complexity of real life you can't solve the maths, and you have to use approximations, and you aren't always sure that the approximations are valid either. Still, where the maths works well, it's really, really, really, really useful.QuoteThe other way to do it is not to use true calculus, but to use small deltas. So you divide the problem up into small chunks, but you do not take it down to the infinitesimal limit. If the distances between the chunks are small enough, this approximates to using calculus. That's done rather a lot, it's called 'finite element analysis' and similar names.If a human can work out exact answers using calculus, why would a machine not solve problems the same way? If a human can do it, it ought to be dead easy for a machine. Maybe it's faster to do it with approximations and that might matter in something like graphics for computer games where precise answers may be unnecessary.
Post by: wolfekeeper on 03/02/2012 22:33:53
You take the limit of the gradient as the line length tends to zero, and you get the answer; that's the gradient at that point.Limits are answers where you find that the answer gets closer and closer to something, without ever quite getting there, (if it actually got there the maths usually explodes, but that doesn't matter!!) But not every equation tends to a limit, some don't converge to a limit at all, and some give you a different answer depending on which side of the limit you start from.
I thought the whole purpose of calculus was the it could get to an exact answer at some point rather than just getting a good approximation. For example, if you're trying to calculate the slope of a curve through a point, you can calculate the slope of a line and make that line shorter and shorter to get a closer and closer approximation of the slope at the point, but you can't do anything useful with a line of zero length. I was led to believe that calculus would somehow enable you to get an exact value for the slope at a point, but I never saw any evidence that this was actually possible during hundreds of hours of watching a teacher fail to explain how calculus was meant to be done.
The answer is exact, the limit of something (when it's soluble) is an EXACT answer, it's not approximate.
The approximation gets better and better as the line length gets shorter, and the limit is precise.
1+1/2+1/4+... the limit of that is 2. It's exactly 2. Likewise the limit of the gradient is the actual gradient at that point (by definition- they define it that way!!)
How do we know it's right? That the gradient really is that? Probably, mathematicians don't, really. But they find that the answers always make sense, they're always self consistent, so it doesn't matter. But really it's pretty obvious that it ought to work.
Post by: David Cooper on 03/02/2012 23:45:42
It would be helpful if this stuff was taught by retaining words in the early stages so that you can remember what things are meant to be. Why not just write dist/time?Because if you do dist/time, that's actually the average speed.
Then so is dx/dy. If the d has some function other than being a constant, wouldn't it be better to have a special symbol for whatever it's supposed to be to avoid confusion? What does the d actually mean?
Quote
What you're trying to calculate is the speed at a particular instant in time. For example, a car that is accelerating may travel 100 metres in 10 seconds, but it wasn't going at 10m/s the whole way. At the beginning it was stationary, at the end it was going at (say) 20 m/s.
How would you work out the speed at the end of the course, if it wasn't accelerating completely uniformly?
I probably couldn't, but I can imagine that there might be a way to do it if you had a formula to describe the acceleration.
Quote
QuoteAnd why does it suddenly go Greek with delta replacing d? The trouble I have with calculus is may be mainly down to a teacher introducing symbols and never restating what they're supposed to mean.Delta means it's not a limit, it's a real number you could type into your calculator, like 0.0001, it's just a small number.
I should have asked what the d meant first and then asked what it meant to replace it with delta.
Quote
No, it doesn't decode them, it treats them as squiggles and messes around with the squiggles. It doesn't have a clue what the squiggles really mean.
At some point it has to take numbers in and at some point it has to produce answers as numbers, and in between those points it has to do something that I've never seen set out clearly, and some of that will involve crunching numbers. I have no doubt I could write a program to do it all too, but I have never seen anyone set out a real problem and illustrate how to solve it in rational steps. All I've ever seen is a teacher repeatedly assert that something can be done and then repeatedly fail to demonstrate how.
You take the limit of the gradient as the line length tends to zero, and you get the answer; that's the gradient at that point.
The answer is exact, the limit of something (when it's soluble) is an EXACT answer, it's not approximate.
The approximation gets better and better as the line length gets shorter, and the limit is precise.
1+1/2+1/4+... the limit of that is 2. It's exactly 2. Likewise the limit of the gradient is the actual gradient at that point (by definition- they define it that way!!)
I can see that it's exactly 2 in this case, but I can't see a process that would enable a machine to work that out. You can add up lots of smaller and smaller fractions and see that it appears to be heading for 2, but if calculus doesn't involve a guess at some stage, there needs to be some point at which the exact answer 2 comes out of an actual calculation.
Quote
How do we know it's right? That the gradient really is that? Probably, mathematicians don't, really. But they find that the answers always make sense, they're always self consistent, so it doesn't matter. But really it's pretty obvious that it ought to work.
With that example (can't see any calculus in it) you can calculate the 2 easily enough through reasoning and see that the total would be exactly 2 if you could add an infinite number of fractions with each half the size of the one before, but in most cases I can't see that it would be possible to reason your way to an exact answer so easily.
Post by: wolfekeeper on 04/02/2012 00:02:14
It means you've taken delta x/delta y to the infinitesimally small limit.It would be helpful if this stuff was taught by retaining words in the early stages so that you can remember what things are meant to be. Why not just write dist/time?Because if you do dist/time, that's actually the average speed.
Then so is dx/dy. If the d has some function other than being a constant, wouldn't it be better to have a special symbol for whatever it's supposed to be to avoid confusion? What does the d actually mean?
Quote
Yes, Ok, if I give you the acceleration, but what if I give you an equation of its position:QuoteWhat you're trying to calculate is the speed at a particular instant in time. For example, a car that is accelerating may travel 100 metres in 10 seconds, but it wasn't going at 10m/s the whole way. At the beginning it was stationary, at the end it was going at (say) 20 m/s.
How would you work out the speed at the end of the course, if it wasn't accelerating completely uniformly?
I probably couldn't, but I can imagine that there might be a way to do it if you had a formula to describe the acceleration.
x = 0.5 t^3 + 3 t^2
what speed is it going at, at time t=10?
Post by: Geezer on 04/02/2012 01:18:39
x = 0.5 t^3 + 3 t^2
what speed is it going at, at time t=10?
Oh dear! It's been a while. Without looking up anything on the internet, or cracking a book
x = 0.5 t^3 + 3 t^2
so,
dx/dt = 1.5 t^2 + 6 t
(probably wrong!)
Post by: David Cooper on 04/02/2012 22:39:03
Yes, Ok, if I give you the acceleration, but what if I give you an equation of its position:
x = 0.5 t^3 + 3 t^2
what speed is it going at, at time t=10?
I wouldn't know where to start with that.
dx/dt = 1.5 t^2 + 6 t
I can do differentiation and integration without any difficulty, but I've never been shown how to apply them to any kind of meaningful problem. Like I said before, doing this stuff at school was like being made to learn a language without ever being allowed to know what any of the words mean, so I've got the algorithms in my head (or at least some of them), but I haven't a clue what can be gained by applying them to anything.
Post by: wolfekeeper on 04/02/2012 23:29:20
If the delta is made very small, then you're averaging the speed over a very small time, then the average speed tends to the limit of the actual, instantaneous speed.
In other words for the exact speed, rather than just the close average, you want dx/dt which is the limit of delta-x/delta t.
So dx/dt is the speed. Geezer already calculated that (correctly!) for you.
So all you have to do is plug t=10 into Geezer's equation, and you have the exact speed at that moment.
Post by: Geezer on 05/02/2012 06:05:17
I poked around a bit and came up with this http://www.youtube.com/watch?v=-duE_JQmjq8 (http://www.youtube.com/watch?v=-duE_JQmjq8)
This lady has gone to some trouble to explain an example in practical terms. (I confess I didn't sit through the entire lecture.)
Post by: CliffordK on 05/02/2012 11:44:38
Sometimes it is easiest to just do a numerical estimate which can be pretty easy to setup with a spreadsheet.
However, it is a good point that one can use derivatives and integrals to convert between distance, velocity, and acceleration.
A few days ago, there was a question...
Say one put a tunnel through the moon, and had magnetic acceleration, how fast could one get an object going.
I came up with an answer numerically with Opencalc, but I see that I should have done it mathematically if I wasn't a bit rusty.
Say we start with a constant Acceleration=10g
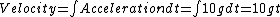
So, now if I set my distance to be the diameter of the moon (3,475 km = 3,475,000 m) and solve for T, I get:
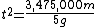
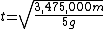
So, t=266.3 seconds.
Plugging that back into the velocity equation, one gets
v=10gt = 10*9.8*266.3 = 26097 m/s = 26 km/s
Now, let's see how I did.
Oops, I did the calcs for 100g (or 1000 m/s2) (http://www.thenakedscientists.com/forum/index.php?topic=42938.msg379155#msg379155)
But, it isn't a big deal... just change my 5g to 50g above.
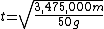
And, I get t=84.2 seconds (or 83.4 seconds if I used g=10m/s2)
V=100gt = 82.5 km/s
Anyway, so essentially my numerical estimate was the same.
But, perhaps not as elegant as using a few squiggles. [;)]
Anyway, you can use calculus to change from distance to velocity to acceleration, and visa versa.
Derivatives gives one slopes (or instantaneous rate of change) of a curve.
Integrals gives one the area under a curve.
Certainly it can be applied to many other things such as population models.
Post by: David Cooper on 06/02/2012 01:05:44
Original equation for the position (distance travelled): x = 0.5 t^3 + 3 t^2
It now appears that differentiating that gives the equation for the speed: dx/dt = 1.5 t^2 + 6 t
(For anyone reading this who doesn't know how differentiation is done, you multiply the 0.5 by the 3 in "^3" [which means cubed] and take 1 away from that 3, then multiply the later 3 by the 2 in "^2" [which means squared] and take 1 away from that 2 [to get "^1" which obviously doesn't need to be stated]. It's a startlingly simple process which I spent months doing in school without ever being allowed to know why.)
Um. Well delta x/delta t is the average speed over a time delta t. So for example delta t might be .1 second and the distance travelled might be 5 metres so the average speed would be 50 m/s.
As soon as these deltas come into it, I get confused because they seem to have no purpose - all it's actually saying is that distance divided by time = average speed.
Quote
If the delta is made very small, then you're averaging the speed over a very small time, then the average speed tends to the limit of the actual, instantaneous speed.
Which it appears will always be zero because you're hacking it back to the speed at the start when it wan't moving.
Quote
In other words for the exact speed, rather than just the close average, you want dx/dt which is the limit of delta-x/delta t.
But here you apply the trick of differentiating the original equation to derive one to give the speed at any point in time - all you need to do then is put in a value for the time and solve it. That's dead easy, but you do have to know that this new equation will serve that purpose. Up until today, I didn't.
Quote
So dx/dt is the speed. Geezer already calculated that (correctly!) for you.
So all you have to do is plug t=10 into Geezer's equation, and you have the exact speed at that moment.
I did that, and since you've confirmed that this is the right way to do things, I now know something that I should have been told at school. Thank you for filling in a massive hole in my education. I have finally seen a practical application for calculus, assuming that this is actually calculus (seems too simple).
Post by: David Cooper on 06/02/2012 01:13:41
I poked around a bit and came up with this http://www.youtube.com/watch?v=-duE_JQmjq8 (http://www.youtube.com/watch?v=-duE_JQmjq8)
This lady has gone to some trouble to explain an example in practical terms. (I confess I didn't sit through the entire lecture.)
I'll watch that next time I travel to somewhere with a fast enough internet connection. I'm stuck with something that keeps jumping between 3G and GPRS and struggles to download audio.
Post by: David Cooper on 06/02/2012 02:17:53
Say we start with a constant Acceleration=10g
So, now if I set my distance to be the diameter of the moon (3,475 km = 3,475,000 m) and solve for T, I get:
So, t=266.3 seconds.
Plugging that back into the velocity equation, one gets
v=10gt = 10*9.8*266.3 = 26097 m/s = 26 km/s
I'm having trouble following that, partly because I can't tell whether letters are constants or units, and I don't know what the dt is doing.
Quote
Anyway, you can use calculus to change from distance to velocity to acceleration, and visa versa.
Well, I can now go from the first to the second and back, but how do you deal with acceleration?
Quote
Derivatives gives one slopes (or instantaneous rate of change) of a curve.
Integrals gives one the area under a curve.
Stated in that form with nothing to tie it to a practical application, that just goes in one ear and out the other. That is why so many people fail to learn calculus - it needs to be grounded in practical application first before it goes abstract. You need to have an understanding of what use it is to work out the area under a curve, and all of these basic things need to be constantly available somewhere for you to refer back to so that you can fix and refix it in your mind. Some teachers fling ideas out at a hundred miles an hour and never repeat them, and you can never tell what's important and what's just a passing comment. There should be a standard map of all this stuff which has the whole thing set out clearly and consicely so that it's easy to see what calculus actually is and what it's for. I'd like to create such a map, but I obviously don't know what to put in it yet.
Quote
Certainly it can be applied to many other things such as population models.
If anyone reading this has applied calculus to a real problem that would further help illustrate its uses, please feel free to share it here - it strikes me that the process is not at all difficult, but that the real problem is understanding what the squiggles mean and knowing what use it is when you apply it to something. (That last sentence is not faulty - you have to read "use" as "yooss" rather than"yooz".)
Thanks for all the help so far - you have already unlocked a door for me, and I hope others are finding this helpful too.
Post by: Geezer on 06/02/2012 03:05:49
It's a startlingly simple process which I spent months doing in school without ever being allowed to know why.
Try this:
A population of bacteria squares every second. Let's call the population p, and the time t.
at time 1, p=1
at time 2, p=4
at time 3, p=9
(you can see where this is going)
If I have not mucked this up, p = t^2
You can draw a graph of what this might look like, but it should be obvious that the slope gets steeper with time. In fact, after a few seconds, the slope is almost vertical.
Differentiation allows you to determine just how steep a slope is at a particular point on the slope. If you plot this example on to a piece of graph paper, you will find that, for example, at time 3 seconds, the slope ratio is 9:1.5 which equals 6.
If you differentiate p = t^2 you get,
dp/dt = 2t
When t is 3, 2t = 6
It's obvious by inspection that the slope ratio is 9:1.5, but I haven't a clue why that is!
Post by: CliffordK on 06/02/2012 04:00:55
I'm having trouble following that, partly because I can't tell whether letters are constants or units, and I don't know what the dt is doing.
Ahh, yes,
I always hate it when letters are poorly defined.
Actually, in the equations I wrote, the only real variable is t = time.
g is the gravitational constant, 9.8 m/s2, where m is meters, and s is seconds. km would be kilometers.
So, like pi, (π), g does not vary, although sometimes I'll round it to 10 m/s2. Your gravity might vary a little based on your location, for example on the moon. But, then it can be written as a fraction of Earth's gravity, g.
m = meters
s = seconds
km = kilometers
I am expressing Distance as a function of time Distance(t).
Likewise Velocity is a function of time Velocity(t).
And Acceleration is a function of time Acceleration(t).
d.... That signifies change. in something. You will also see delta (Δ), which means change in time, or the symbol (∂) for partial derivatives.
Why the dt? I suppose it tells you that you are evaluating a function with respect to the change of time. And, thus, it is required to be a part of integration.
Hmmm
Relating Distance, Velocity, and Acceleration.
if f(t) is a distance function with respect to time t.
Then Velocity(t) is a function of the rate of change of the distance at any time t.
I.E. If you are driving from LA to New York. If Velocity = 0, that means you are parked, and distance is not changing.
If the Velocity is > 0, then you are going forward.
If the Velocity is < 0, then you are going backward.
Anyway, the Velocity(t) is the rate of change of the distance function, or the derivative of the distance function d(f(x))
Acceleration tells you how fast you are changing the velocity function (how much you've pressed the gas pedal down). So, it would be the slope of the velocity function at any given time. Acceleration(t) = d(Velocity(t)) = d(d(f(t))
I suppose I forgot that when one takes derivatives, one looses one's constant factors. So, taking integrals, one should add them back in when doing integrals. I.E. What is the initial distance or velocity, which in some cases can be set to zero.
Anyway, the mathematics is relevant for scientific discussions. However, one can often understand a lot about a subject without needing anything very complex.
Post by: David Cooper on 06/02/2012 05:56:45
Try this:
A population of bacteria squares every second. Let's call the population p, and the time t...
Differentiation allows you to determine just how steep a slope is at a particular point on the slope.
I managed to follow that. The slope of the graph doesn't seem so immediately meaningful in human terms, unlike in a slope in a graph where the slope relates to the speed of travel (which is something that we can relate to well), but yes: it will be the speed of population growth at that point (if that really is how a population of something grows, though that doesn't seem likely - doubling every so many minutes/hours would I'm guessing be more realistic for bacteria, and with other kinds of creature it might follow the Fibonacci Sequence).
By the way, this online graphic calculator might come in handy if anyone needs to see what any of these equations looks like on a graph (it uses Java):-
http://www.coolmath.com/graphit/ (http://www.coolmath.com/graphit/)
Post by: David Cooper on 06/02/2012 07:10:26
Velocity = acceleration x time, but here you're complifying it by putting
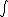in front and turning time into dt, then you remove the 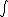and the d disappears. I don't know what the rule is that allows you to do this, but it doesn't look all that simple as it gets more complicated the next time:-Quote
Distance is the area under the graph if the graph is of the velocity, so I can see the reason for the
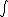in front of velocity this time. I'm trying to work out what the dt bit means here, but clearly it can't just be velocity on its own as it needs to be an equation of the velocity, so that appears to be the meaning of the dt. Where I then get lost is where the 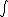disappears and appears to take the dt with it instead of just the d as it did last time. This kind of manipulation of parts of equations is another area which my maths teacher failed to put across in anything remotely resembling a systematic way - she did it without explanations and made it seem like witchcraft.if f(t) is a distance function with respect to time t.
Straight away I feel ill - this is caused by a year of seeing "f(x)=" appearing all over the board for no obvious reason. It left me with a complex about it and anything that looks like it because it was never meaningful. This is why I'd like to ban all the squiggles in the early stages and do as much as possible using normal words.
Quote
Then Velocity(t) is a function of the rate of change of the distance at any time t.
I.E. If you are driving from LA to New York. If Velocity = 0, that means you are parked, and distance is not changing.
If the Velocity is > 0, then you are going forward.
If the Velocity is < 0, then you are going backward.
Had to read that a couple of times, but then it clicked.
Quote
Anyway, the Velocity(t) is the rate of change of the distance function, or the derivative of the distance function d(f(x))
If f(x) causes me problems (it makes my mind go blank), imagine what d(f(x)) does! I won't be able to handle these shorthands for things until I've understood it in normal language. It's hard enough when it's still in normal language without wanting to remove that and replace any of it with squiggles.
Quote
Acceleration tells you how fast you are changing the velocity function (how much you've pressed the gas pedal down). So, it would be the slope of the velocity function at any given time. Acceleration(t) = d(Velocity(t)) = d(d(f(t))
And that's even worse. If you're taught this stuff the right way from the outset it may be easy, but if you've been taught it badly and have nightmares about it, there's a lot of damage that needs to be undone. I just can't handle the squiggles at this stage. The maths behind it all is clearly dead easy, but it's all being hidden behind a wall of squiggles which only get in the way of understanding. These squiggles are a shorthand for mathematicians to make things more complact - they are not appropriate as a way in.
Quote
I suppose I forgot that when one takes derivatives, one looses one's constant factors. So, taking integrals, one should add them back in when doing integrals. I.E. What is the initial distance or velocity, which in some cases can be set to zero.
I understand that without difficulty though.
Quote
Anyway, the mathematics is relevant for scientific discussions. However, one can often understand a lot about a subject without needing anything very complex.
Indeed one can, but there's no reason why anyone should be shut out of the maths - it is simplicity obscured by squiggles. What people really need to see to get an easy ride into calculus (or anything else of this kind) is clear rules laid out as to how to go about things, clear descriptions of what things are, and clear demonstrations of the procedures you have to go through to solve problems. If any of the tools aren't available to you or if any part of the language isn't clear to you, you're stuffed - you can appear to be a failure at maths, and yet that is not the case. The real problem is that you haven't been given a checklist of tools so that you can be sure you're working with the complete set, you haven't been given an adequate (or even an inadequate) manual explaining the squiggles which you can go back to consult at any time, and you're pushed into doing everything through squiggles before you're ready. It's no wonder that so many people get stuck here (or somewhere else in maths) and never get any further. The problem isn't with the learners, but with the teaching.
Post by: Geezer on 06/02/2012 08:24:16
Indeed one can, but there's no reason why anyone should be shut out of the maths - it is simplicity obscured by squiggles. What people really need to see to get an easy ride into calculus (or anything else of this kind) is clear rules laid out as to how to go about things, clear descriptions of what things are, and clear demonstrations of the procedures you have to go through to solve problems. If any of the tools aren't available to you or if any part of the language isn't clear to you, you're stuffed - you can appear to be a failure at maths, and yet that is not the case. The real problem is that you haven't been given a checklist of tools so that you can be sure you're working with the complete set, you haven't been given an adequate (or even an inadequate) manual explaining the squiggles which you can go back to consult at any time, and you're pushed into doing everything through squiggles before you're ready. It's no wonder that so many people get stuck here (or somewhere else in maths) and never get any further. The problem isn't with the learners, but with the teaching.
I think you are correct. Academia has no excuse for failing to make calculus as easy to understand as arithmetic.
Post by: RD on 06/02/2012 12:34:58
some people are born bad at maths ... http://en.wikipedia.org/wiki/Discalculia
Post by: Geezer on 07/02/2012 01:07:22
Post by: David Cooper on 07/02/2012 21:41:22
Post by: wolfekeeper on 07/02/2012 21:51:26
Nearly, it's the distance CHANGE and the time CHANGE.Um. Well delta x/delta t is the average speed over a time delta t. So for example delta t might be .1 second and the distance travelled might be 5 metres so the average speed would be 50 m/s.
As soon as these deltas come into it, I get confused because they seem to have no purpose - all it's actually saying is that distance divided by time = average speed.
distance change divided by time change = average speed over that time
i.e.
delta x / delta t = average speed
Quote
Wrong!!!! And it's wrong in an important way.QuoteIf the delta is made very small, then you're averaging the speed over a very small time, then the average speed tends to the limit of the actual, instantaneous speed.
Which it appears will always be zero because you're hacking it back to the speed at the start when it wan't moving.
These are DELTAS so it would be delta x (0) over delta t (0) = 0/0 = undefined
So you can't put it all the way back, because the maths blows up when you set the time delta to exactly zero, a zero sized triangle has no calculable gradient.
But by doing the LIMIT, you are effectively setting the time delta an infinitesimally small amount above zero, so it doesn't blow up, and you get a sensible answer. And that's how calculus works.
Post by: RD on 07/02/2012 22:22:58
At the risk of hopelessly derailing the thread (not that that ever stopped me) I saw something recently about people seeing numbers in particular colo(u)rs, at least I think it was numbers.
(https://upload.wikimedia.org/wikipedia/commons/thumb/6/60/Synesthesia.svg/250px-Synesthesia.svg.png) (https://en.wikipedia.org/wiki/Synesthesia)
Post by: David Cooper on 07/02/2012 22:26:22
Q. Are some people born good at maths?
some people are born bad at maths ... http://en.wikipedia.org/wiki/Discalculia
There's been a heated argument about dyslexia with some people claiming there's no such condition - I saw a documentary about this where they took dyslexic children and taught them how to read properly, thereby curing them completely and in a very short time. I wouldn't be surprised if the same argument is going on with dyscalculia, even though with both conditions there appears to be quite a weight of evidence to support them. The truth may be that most examples of people diagnosed with these conditions don't actually have them, but some may well do.
There are also very different ways of learning the basics of maths, and some are better than others - one of the biggest difficulties comes up right at the start and it is not understood by most teachers. When you add two numbers together you have to do two lots of counting at the same time. Take 5 + 4 as an example: you have to count up from the 5 as you add on the 4, but you also have to count up to 4 so that you know when you've added it all on (start at 5...; 6, 1; 7, 2; 8, 3; 9, 4: the answer's 9). That isn't easy, and children are left to work out their own ways of doing it. Many of them do one of the counts on their fingers, usually the wrong one (which limits them to answers under 11). When I was 4 (and before I'd started school) I came up with a method based on rhythm where each number being added or taken away had a rhythm associated with it which would run out at the point when I'd counted up to the answer. Once at school, I came up with another method which enabled me to do long boring sums without error and without having to think, this time tapping the numbers with the point of a pencil while counting (each digit having a different pattern of dots imagined onto it) - it isn't as quick as doing it from memorised answers, but it's so reliable that it eliminates the need to check your answers and ensures that you get everything 100% right. These methods aren't taught, so children are left to find their own way to do the two lots of counting at once, and some of them never do manage to find a good way. The usual idea is that children should simply practise again and again until they eventually memorise the answers and the little tricks for getting past boundaries where the tens are affected, but some get to that stage years ahead of others because they have better ways of working them out in the first place - the rest are often memorising wrong answers and methods and tying themselves in knots as a result. Far too much is left to chance - if you want to ensure that children to be able to do maths you need to make sure that there are no gaps in their knowledge and that they have the full set of tools available to them. Most children miss something important in maths at some point, and then they're forced to try to build on top of an incomplete foundation without being given any opportunity to get hold of the missing piece(s). This makes if very hard to untangle whether any individual is innately bad at maths or if they just have a gap/gaps in their knowledge or a dodgy method for doing something which throws them off balance and makes them feel sick whenever they set eye on a piece of mathematics.
Post by: David Cooper on 07/02/2012 22:29:51
(https://upload.wikimedia.org/wikipedia/commons/thumb/6/60/Synesthesia.svg/250px-Synesthesia.svg.png) (https://en.wikipedia.org/wiki/Synesthesia)
I wonder how they feel when looking at something like that if the colours are wrong for them.
Post by: David Cooper on 07/02/2012 23:44:23
As soon as these deltas come into it, I get confused because they seem to have no purpose - all it's actually saying is that distance divided by time = average speed.Nearly, it's the distance CHANGE and the time CHANGE.
distance change divided by time change = average speed over that time
i.e.
delta x / delta t = average speed
But it isn't a change in distance - it's a change in location, and the distance represents the amount of change in location. With "time" it's more ambiguous as you can see time both as a location and as a duration, the latter being a change of the former. So, it now looks to me as if it's saying distance/duration, or change in location / change in time-location. Either way, I still can't see why the delta is necessary because metres and seconds are primarily units of distance and duration rather than locations.
Quote
QuoteWrong!!!! And it's wrong in an important way.QuoteIf the delta is made very small, then you're averaging the speed over a very small time, then the average speed tends to the limit of the actual, instantaneous speed.
Which it appears will always be zero because you're hacking it back to the speed at the start when it wan't moving.
These are DELTAS so it would be delta x (0) over delta t (0) = 0/0 = undefined
So you can't put it all the way back, because the maths blows up when you set the time delta to exactly zero, a zero sized triangle has no calculable gradient.
But by doing the LIMIT, you are effectively setting the time delta an infinitesimally small amount above zero, so it doesn't blow up, and you get a sensible answer. And that's how calculus works.
My point was with that example simply that you can see from the graph that if you make it infinitely small the speed will be zero - it doesn't give you any way to calculate the speed at a more interesting point on the graph. Since then we've got beyond that and I've seen that you can derive a new graph from the original one and then pick any time you like, replcae t with it, solve the equation and out pops the precise speed for that point in time.
Post by: wolfekeeper on 08/02/2012 00:20:29
But it isn't a change in distance - it's a change in location, and the distance represents the amount of change in location.For example at t=3, you might be at x=4, 4 is a distance from the origin
and at t=3.3 you might be at 4.2, 4.2 is the distance from the origin
But delta x is NOT 4 or 4.2, no, it's the change in distance, the distance delta (delta literally just means change) in the distance: 4.2 - 4 = 0.2 = delta x when you make a small step in time
Quote
Either way, I still can't see why the delta is necessary because metres and seconds are primarily units of distance and duration rather than locations.They're also units of change/delta of distance and duration.
Post by: David Cooper on 08/02/2012 21:10:48
But it isn't a change in distance - it's a change in location, and the distance represents the amount of change in location.For example at t=3, you might be at x=4, 4 is a distance from the origin
and at t=3.3 you might be at 4.2, 4.2 is the distance from the origin
But delta x is NOT 4 or 4.2, no, it's the change in distance, the distance delta (delta literally just means change) in the distance: 4.2 - 4 = 0.2 = delta x when you make a small step in time.
Well, it's a very strange way of describing something - the 0.2 is itself a distance between two locations, so to call it a change in distance seems unnecessary unless you're thinking about actively changing the distance between two points in some way by moving one or both of them along the graph.
Post by: wolfekeeper on 08/02/2012 22:10:34
Post by: Geezer on 08/02/2012 23:16:21
It's not just any distance, it's specifically a very small distance, as small as possible.
Right! You really could care less what the actual distance is because you are only interested in the ratio of the distances in two axes.
Perhaps you could substitute something along the lines of "the ratio of the axes at any point" for the shorthand "dx/dy"
Post by: imatfaal on 09/02/2012 12:01:15
Technically , dy/dx is not a ratio - it is the limit or just a notation. Mad mathematicians would tell you that the ratio of two infinitessimals can be anything you choose (kind of like the ration of two infinities it is not well defined). It is a technical point and not that important - I have seen thinking of dy/dx as a ratio described as a "useful crutch that could get you into difficulties later". The reasons are abstruse and arcane - and I couldn't rehearse them off hand (or possibly at all), but I have followed some very heated exchanges in which mathematicians lose their rag trying to explain to the math forum visitor why it should not technically be thought of as ratioIt's not just any distance, it's specifically a very small distance, as small as possible.
Right! You really could care less what the actual distance is because you are only interested in the ratio of the distances in two axes.
Perhaps you could substitute something along the lines of "the ratio of the axes at any point" for the shorthand "dx/dy"
Post by: wolfekeeper on 09/02/2012 14:48:17
Technically , dy/dx is not a ratio - it is the limit or just a notation.Yes, that's correct, although if you ignore that, it turns out that you largely get away with it.
Quote
Mad mathematicians would tell you that the ratio of two infinitessimals can be anything you choose (kind of like the ration of two infinities it is not well defined).No, AFAIK that's definitely wrong. The reason that calculus works is that the LIMIT of dy/dx as you take the deltas towards zero is (in most normal cases) completely well defined. In some case limits can take two values in different directions where the curve jumps, so the curve has to be smooth where you differentiate; trying to differentiate a fractal doesn't do anything very good!
It's the number you get when you divide two numbers that are actually zero which is undefined, not the limit.
Quote
It is a technical point and not that important - I have seen thinking of dy/dx as a ratio described as a "useful crutch that could get you into difficulties later". The reasons are abstruse and arcane - and I couldn't rehearse them off hand (or possibly at all), but I have followed some very heated exchanges in which mathematicians lose their rag trying to explain to the math forum visitor why it should not technically be thought of as ratioYeah, probably, dy/dx is really a notational thing, and there's some spectacular ways you can get into trouble with not realising this. Cancelling the 'd's is the least of your problems if you don't realise it(!) Also d^2y/dx^2 is even more obviously different. You can at least multiply dy/dx by dx and it makes sense and gives you dy, whereas multiplying d^2y/dx^2 by stuff doesn't do anything very good at all so far as I recall.
Post by: Geezer on 09/02/2012 16:05:37
Post by: imatfaal on 09/02/2012 17:38:07
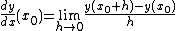
Maybe I didnt explain well - but it is not wrong. There is a big difference between the limit as I have it above and the ratio of two variables as they tend to zero. dx is not theMad mathematicians would tell you that the ratio of two infinitessimals can be anything you choose (kind of like the ration of two infinities it is not well defined).No, AFAIK that's definitely wrong. The reason that calculus works is that the LIMIT of dy/dx as you take the deltas towards zero is (in most normal cases) completely well defined. In some case limits can take two values in different directions where the curve jumps, so the curve has to be smooth where you differentiate; trying to differentiate a fractal doesn't do anything very good!
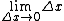as this is zero.
Post by: Geezer on 09/02/2012 17:57:35
yes - but when you deal with limits approaching zero or off to infinity things become very complicated - instantaneous measurements calculations etc can become very screwy .
WHOOSH!!
(that was the sound of your post zooming over my head)
I think I better stick to uppyness and alongyness.
Post by: Geezer on 09/02/2012 18:46:00
These points are interesting to the mathematically inclined, but to the average engineer who just wants to solve a stinking problem, they might appear slightly esoteric and academic.
It's like any tool. The experts will test it to the limits and understand all of its corner-cases. The rest of us will use it like any other hammer, and, if it doesn't work, we'll get a bigger one.
Post by: JP on 10/02/2012 02:43:45
You can use it without worrying too much, but sometimes...
(By the way, as a physicist I'm very guilty of using derivatives as fractions and throwing infinity around with reckless abandon.)
Post by: imatfaal on 10/02/2012 09:48:31
Actually, isn't this, to some extent, what the OP was referring to?
These points are interesting to the mathematically inclined, but to the average engineer who just wants to solve a stinking problem, they might appear slightly esoteric and academic.
It's like any tool. The experts will test it to the limits and understand all of its corner-cases. The rest of us will use it like any other hammer, and, if it doesn't work, we'll get a bigger one.
Almost totally agree - but with same proviso as JP. The hard core scientists I know are all very clear on the rules and derivation of those rules and then break them almost all the time - I swear I once saw someone cancelling the ds in dy/dx
Post by: Geezer on 11/02/2012 21:48:26
Post by: sasha44 on 12/02/2012 05:23:48