1
This section allows you to view all posts made by this member. Note that you can only see posts made in areas you currently have access to.
2
Physics, Astronomy & Cosmology / Re: Is there a substance where entropy decreases at higher temperature?
« on: 16/12/2022 05:51:33 »
Hi.
I've had a bit of time to think. As you ( @chiralSPO ) implied: It's very much a case of needing to keep our definitions of temperature straight (and it does become a little dull then).
The situation with a simple two-energy level system does illustrate a situation where temperature can increase (in this case it is increasing by becoming less negative) while the entropy decreases. As you ( @chiralSPO ) implied it's clear that energy is always being added to the system as more particles are driven to the higher energy state, however that wouldn't guarantee that the temperature was increasing.
For a typical substance like an ideal gas held at constant volume, it's apparent that increasing the internal energy will increase the temperature. For a more arbitrary substance and where statistical temperature is used, you really do have to directly check the gradient ∂S/∂E. A diagram will save 1000 words here:
Suppose the entropy, S, of a system of N particles (each particle having only two energy levels) varied with E, the total energy of the system, as follows:

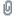 Untitled.jpg (49.3 kB . 1152x648 - viewed 2374 times)
Untitled.jpg (49.3 kB . 1152x648 - viewed 2374 times)
An increase in the the total energy of the system would not produce an increase in temperature once you've entered the region with most of the particles in the higher energy state. The temperature is negative in that region but perfectly constant.
Fortunately the entropy of the simple 2-energy level system doesn't look like that, it has this sort of shape:

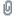 entropy2.png (24.19 kB . 882x680 - viewed 2455 times)
entropy2.png (24.19 kB . 882x680 - viewed 2455 times)
Where the gradient = 1/T is monotonically decreasing with E.
= 1/T is monotonically decreasing with E.
With that established: The simple 2-energy level system does have the usual relationship where increasing its energy remains synonymous with increasing its temperature. The note of caution in your ( @chiralSPO ) first sentence is then substantially laid to rest:
More generally, the simple 2-energy level system ticks all the boxes that I was asking for in the original post. So, well done and thank you. You can have the best answer award.
-------
I don't think I need to ask anything else. If you restrict attention to just non-negative temperatures, then I'm now fairly sure that the answer rests entirely upon finding a substance with specific heat capacity, CV < 0.
While I don't know of any substance with that property, in any situation (e.g. in any range of temperature, pressure or other conditions), if someone else does - Please let me know.
Best Wishes.
I've had a bit of time to think. As you ( @chiralSPO ) implied: It's very much a case of needing to keep our definitions of temperature straight (and it does become a little dull then).
The situation with a simple two-energy level system does illustrate a situation where temperature can increase (in this case it is increasing by becoming less negative) while the entropy decreases. As you ( @chiralSPO ) implied it's clear that energy is always being added to the system as more particles are driven to the higher energy state, however that wouldn't guarantee that the temperature was increasing.
For a typical substance like an ideal gas held at constant volume, it's apparent that increasing the internal energy will increase the temperature. For a more arbitrary substance and where statistical temperature is used, you really do have to directly check the gradient ∂S/∂E. A diagram will save 1000 words here:
Suppose the entropy, S, of a system of N particles (each particle having only two energy levels) varied with E, the total energy of the system, as follows:
An increase in the the total energy of the system would not produce an increase in temperature once you've entered the region with most of the particles in the higher energy state. The temperature is negative in that region but perfectly constant.
Fortunately the entropy of the simple 2-energy level system doesn't look like that, it has this sort of shape:
Where the gradient
 = 1/T is monotonically decreasing with E.
= 1/T is monotonically decreasing with E.With that established: The simple 2-energy level system does have the usual relationship where increasing its energy remains synonymous with increasing its temperature. The note of caution in your ( @chiralSPO ) first sentence is then substantially laid to rest:
I think you need to be careful about specifying whether you mean temperature or thermal energy.
More generally, the simple 2-energy level system ticks all the boxes that I was asking for in the original post. So, well done and thank you. You can have the best answer award.
-------
I don't think I need to ask anything else. If you restrict attention to just non-negative temperatures, then I'm now fairly sure that the answer rests entirely upon finding a substance with specific heat capacity, CV < 0.
While I don't know of any substance with that property, in any situation (e.g. in any range of temperature, pressure or other conditions), if someone else does - Please let me know.
Best Wishes.
The following users thanked this post: chiralSPO
3
Physics, Astronomy & Cosmology / Re: Is there a substance where entropy decreases at higher temperature?
« on: 15/12/2022 02:39:37 »
Hi and thanks.
Lasers and population inversion was exactly what I had in the back of my mind.
This sentence
Obviously I'm going to have to take a bit longer to consider everything. Thank you very much for your time and it's exactly the sort of thing I was looking for.
Best Wishes.
Lasers and population inversion was exactly what I had in the back of my mind.
This sentence
One reasonable definition of energy is: T = ∂U/∂S (constant volume).might be a typing error. That's a perfectly good thermodynamic definition of temperature.
Obviously I'm going to have to take a bit longer to consider everything. Thank you very much for your time and it's exactly the sort of thing I was looking for.
Best Wishes.
The following users thanked this post: chiralSPO
4
Chemistry / Re: How can I find the optimum ΔH and ΔS for passive T control?
« on: 01/06/2022 02:39:40 »5
Chemistry / Re: How can I find the optimum ΔH and ΔS for passive T control?
« on: 01/06/2022 02:37:00 »
Hi again.
@chiralSPO - this is what I had started writing but stopped. It's rough, not spell-checked and just not finished. However, if it helps you, then you can see it. You'll realise why I stopped.... it's too long and I started by explaining things too simply, as if other users might also be reading it.
Actually it just is too long.... the forum won't allow me to post anything with too many characters..... I'll split it into two chunks. I'll also put it under a "spoiler" so it won't clog up all the space for people who are reading this thread.
- - - - - - - - - - - -
Chunk 1
---End of Chunk 1 ---
@chiralSPO - this is what I had started writing but stopped. It's rough, not spell-checked and just not finished. However, if it helps you, then you can see it. You'll realise why I stopped.... it's too long and I started by explaining things too simply, as if other users might also be reading it.
Actually it just is too long.... the forum won't allow me to post anything with too many characters..... I'll split it into two chunks. I'll also put it under a "spoiler" so it won't clog up all the space for people who are reading this thread.
- - - - - - - - - - - -
Chunk 1
Spoiler: show
---End of Chunk 1 ---
The following users thanked this post: chiralSPO
6
Chemistry / Re: How can I find the optimum ΔH and ΔS for passive T control?
« on: 30/05/2022 15:25:22 »
Hi again.
I'm already on pages of work. Therefore no-one will want to read it, not even @chiralSPO .
I do not have the time to complete. I'm way behind on several other important tasks. The whole thing is now begining to cause me some stress and that's not how a forum should be.
I'm very sorry @chiralSPO but you should not wait for a detailed response from me, it would safer to assume it won't happen.
Apologies, Eternal Student.
I'll try and get it done but I'm off to the big city tomorrow and I know I won't be writing much here for at least a day.I'm really sorry but I'm not going to get this done any time soon.
I'm already on pages of work. Therefore no-one will want to read it, not even @chiralSPO .
I do not have the time to complete. I'm way behind on several other important tasks. The whole thing is now begining to cause me some stress and that's not how a forum should be.
I'm very sorry @chiralSPO but you should not wait for a detailed response from me, it would safer to assume it won't happen.
Apologies, Eternal Student.
The following users thanked this post: chiralSPO
7
Chemistry / Re: How can I find the optimum ΔH and ΔS for passive T control?
« on: 27/05/2022 09:00:21 »
While it's true that ΔS ≈ ΔH / 300 is pretty much the criterion for optimal regulation at 300K, it is just restating the requirement that the melting point is near 300.
And tables of melting points are easier to find than tables of ΔS
It's also worth thinking about the "units".
As Alan didn't notice, ΔH is typically given per mole or per unit mass.
On a practical basis you might want to look at the value per cubic metre.
And then you need to look at ΔH per £.
And tables of melting points are easier to find than tables of ΔS
It's also worth thinking about the "units".
As Alan didn't notice, ΔH is typically given per mole or per unit mass.
On a practical basis you might want to look at the value per cubic metre.
And then you need to look at ΔH per £.
The following users thanked this post: chiralSPO
8
Chemistry / Re: How can I find the optimum ΔH and ΔS for passive T control?
« on: 27/05/2022 04:40:39 »
Hi again.
I've had another look at the mathematics and tried a few approaches. I've got something that looks reasonable but it's taking too long to write it down and mark up the equations with LaTex. I'll try and get it done but I'm off to the big city tomorrow and I know I won't be writing much here for at least a day.
The final results I can write down. They are dissapointing and probably only tell you what you should have already been able to guess. On the other hand... they make sense and that suggests I haven't gone too far wrong. Glass half-full or half-empty.
1. You want to have as large an amount of Substance A (or equivalently of substance Z) as possible. i.e. Whatever you do, do lots of it - make the biggest system you can.
2. You want a reaction with as large a ΔH as you can get. Infinity would be great but otherwise just as large as you can get.
3. What about ΔS? If you want maximum response from the system at room temperature (say about 300 Kelvin). Then you will need ΔS ≈ ΔH / 300. More generally the system will act most effectively as a buffer for the room temperature when ΔS = (whatever your value of ΔH is) divided by (whatever value of T you're trying to hold the room at). Changing the value of ΔS from that value has a rapid and detrimental effect: The system becomes almost non-changing (and hence unable to buffer the temperature) at your desired room temperature. Instead it works well as a buffer at a different temperature (which will be precisely T = ΔH / ΔS ).
You could have guessed these couldn't you? The mathematics will show it but it's going to be a day or more.
Best Wishes.
I've had another look at the mathematics and tried a few approaches. I've got something that looks reasonable but it's taking too long to write it down and mark up the equations with LaTex. I'll try and get it done but I'm off to the big city tomorrow and I know I won't be writing much here for at least a day.
The final results I can write down. They are dissapointing and probably only tell you what you should have already been able to guess. On the other hand... they make sense and that suggests I haven't gone too far wrong. Glass half-full or half-empty.
1. You want to have as large an amount of Substance A (or equivalently of substance Z) as possible. i.e. Whatever you do, do lots of it - make the biggest system you can.
2. You want a reaction with as large a ΔH as you can get. Infinity would be great but otherwise just as large as you can get.
3. What about ΔS? If you want maximum response from the system at room temperature (say about 300 Kelvin). Then you will need ΔS ≈ ΔH / 300. More generally the system will act most effectively as a buffer for the room temperature when ΔS = (whatever your value of ΔH is) divided by (whatever value of T you're trying to hold the room at). Changing the value of ΔS from that value has a rapid and detrimental effect: The system becomes almost non-changing (and hence unable to buffer the temperature) at your desired room temperature. Instead it works well as a buffer at a different temperature (which will be precisely T = ΔH / ΔS ).
You could have guessed these couldn't you? The mathematics will show it but it's going to be a day or more.
Best Wishes.
The following users thanked this post: chiralSPO
9
Chemistry / Re: How can I find the optimum ΔH and ΔS for passive T control?
« on: 25/05/2022 23:55:43 »
Hi.
This is the conventional equation:
ΔG = RT Ln (Q/K)
Where ΔG = Gibbs free energy change for the system, (in the forward direction and at the specified concentrations).
Q = quotient of concentrations of products / reactants = [Z] / [A]
K = chemical equilibrium constant = Quotient as above but AT EQUILIBRIUM.
Just to clarify this, this ΔG is a function of 3 variables: The temperature, T, and the concentrations [Z] and [A].
[reference: https://chem.libretexts.org/Courses/Grand_Rapids_Community_College/CHM_120_-_Survey_of_General_Chemistry/7%3A_Equilibrium_and_Thermodynamics/7.11%3A_Gibbs_Free_Energy_and_Equilibrium ]
There seems to be a K missing in your expression, much as if you were assuming K always = 1.
This could be enough to stop your idea working completely. If K = 1 always, then the net reaction never shifts forward or backward - the equillibrium point remains with equal concentrations of products and reactants [Z] = [A] regardless of what happens. In this way it won't respond to changes in temperature at all.
To re-phrase this K ≠1. It is essential that K = K(T) = some function of temperature.
Using conventional theory, it seems that we can approximate K(T) = equillibrium constant at temperature T as
K(T) ≈ e -(ΔG°/ RT )
This quantity, ΔG° is not a function of the concentrations of the products and reactants. At most it is a function of the temperature, T, but more usually the temperature and pressure are also assumed to be standard temp. and pressure. Since you're interested in changes occurring around room temp. and pressure, it shouldn't be a problem to assume ΔG° is just a constant which you can find in a book for the reaction A → Z.
Anyway, re-arranging that equation we obtain: ΔG° = -RT Ln (K) = -RT Ln ([Z]/[A]) where [Z] and [A] are now only to be taken as the concentrations at equillibrium. That might have been the equation you were suggesting in your original post. It matters a lot because, if that was what you were doing, then when you re-arranged it to find ΔH I don't think it was the ΔH that you were actually hoping or thinking you'd find.
Summary: Sorry that was confusing. I'm confused and just trying to match up your notation with that used in some other texts on the subject. I need you to check or explain what it was you were hoping to suggest with your formula ΔG = -RT Ln ([Z] / [A]) .
Best Wishes.
This question is inspiredWell, it is quite a good idea.
ΔG = âRTln([Z]/[A])Could you clarify this please? I'm not sure what your ΔG is, is it actually ΔG° ? Are [Z] and [A] concentrations at equillibirum only? i.d.k.
This is the conventional equation:
ΔG = RT Ln (Q/K)
Where ΔG = Gibbs free energy change for the system, (in the forward direction and at the specified concentrations).
Q = quotient of concentrations of products / reactants = [Z] / [A]
K = chemical equilibrium constant = Quotient as above but AT EQUILIBRIUM.
Just to clarify this, this ΔG is a function of 3 variables: The temperature, T, and the concentrations [Z] and [A].
[reference: https://chem.libretexts.org/Courses/Grand_Rapids_Community_College/CHM_120_-_Survey_of_General_Chemistry/7%3A_Equilibrium_and_Thermodynamics/7.11%3A_Gibbs_Free_Energy_and_Equilibrium ]
There seems to be a K missing in your expression, much as if you were assuming K always = 1.
This could be enough to stop your idea working completely. If K = 1 always, then the net reaction never shifts forward or backward - the equillibrium point remains with equal concentrations of products and reactants [Z] = [A] regardless of what happens. In this way it won't respond to changes in temperature at all.
To re-phrase this K ≠1. It is essential that K = K(T) = some function of temperature.
Using conventional theory, it seems that we can approximate K(T) = equillibrium constant at temperature T as
K(T) ≈ e -(ΔG°/ RT )
This quantity, ΔG° is not a function of the concentrations of the products and reactants. At most it is a function of the temperature, T, but more usually the temperature and pressure are also assumed to be standard temp. and pressure. Since you're interested in changes occurring around room temp. and pressure, it shouldn't be a problem to assume ΔG° is just a constant which you can find in a book for the reaction A → Z.
Anyway, re-arranging that equation we obtain: ΔG° = -RT Ln (K) = -RT Ln ([Z]/[A]) where [Z] and [A] are now only to be taken as the concentrations at equillibrium. That might have been the equation you were suggesting in your original post. It matters a lot because, if that was what you were doing, then when you re-arranged it to find ΔH I don't think it was the ΔH that you were actually hoping or thinking you'd find.
Summary: Sorry that was confusing. I'm confused and just trying to match up your notation with that used in some other texts on the subject. I need you to check or explain what it was you were hoping to suggest with your formula ΔG = -RT Ln ([Z] / [A]) .
Best Wishes.
The following users thanked this post: chiralSPO
10
Physiology & Medicine / Re: why is my skin so sensitive when I have a fever?
« on: 19/05/2022 16:14:22 »Is this a known effect?Very much so, especially for flu. It is similar to heightened sensitivity to sound and light, especially when feverish.
Quote
Is there a known (or likely) mechanism?Apparently staying hydrated is a good way to limit it. Ibuprofen helps reduce inflamatory related symptoms, including the skin sensitivity. I found that acetaminophen does a nice job on headaches and fever, but not so helpful with the inflamation.
Is there anything I can do to limit it while recovering?
Benefit of covid: Our altered social practices have seemingly prevented about two years of all the common stuff I/we usually contract each year. Sorry this hasn't been entirely true for you.

The following users thanked this post: chiralSPO
11
General Science / Re: Is 2 really prime? If so, why isn't 1?
« on: 01/05/2022 04:11:50 »
There are also prime polynomials (if you ignore imaginary zeroes).
- These are important in telecommunications and encryption schemes
- These are important in telecommunications and encryption schemes
The following users thanked this post: chiralSPO
12
General Science / Re: Is 2 really prime? If so, why isn't 1?
« on: 30/04/2022 00:19:02 »
Hi.
There is already some terminology you could use to describe a set of things that behave like prime numbers but apply to a much more generalised set of objects than just the Natural numbers. These things are called "prime elements" and the parent algebraic structure is known as a "Ring". You seem to be interested in the Ring which is the Integers (positive and negative Naturals with 0, under conventional binary operations of + and x).
See Wikipedia entry: Prime elements, if you're interested. https://en.wikipedia.org/wiki/Prime_element
However, you should note that they exclude "units" which would include -1 in the ring of Integers, i.e. they would directly exclude both +1 or -1 from the prime elements in the ring of Integers. (For what reason? Similar to excluding 1 from the primes, it makes it much easier to state an equivalent unique factorisation theorem for the ring of Integers).
Here's a quick question or puzzle, just for fun. It relates to the idea you mentioned earlier of eliminating the number 2 from the prime numbers. You also seemed keen to extend beyond the positive numbers and consider negative numbers but you really don't have to stop there - you can consider Complex integers.
The Complex Integers or "Gaussian Integers" are the Complex number equivalents of integers. Specifically, the Gaussian integers are the set of all complex numbers of the form a+bi where a and b are integers.
Just like in ordinary arithmetic with Natural numbers, a prime (or prime element) of the Gaussian integers is a Gaussian integer, p, that is irreducible or cannot be factorised. Specifically, if we have p = q à r (where à is just ordinary multiplication of the complex integers q and r) then at least one of q or r must be a unit element. A "unit" is any complex number that lies on a unit circle around 0, so the only units in the Gaussian integers are +1, -1, +i, -i.
The number 2 is a prime in the ordinary integers. Is it still a prime in the Gaussian integers? To say that another way, can you factorise the number 2 in the Gaussian integers?
Best Wishes.
I came across another wrinkle: what about â1?Your general arguments after this are reasonable. However, I think it is again just a matter of simplicity and having a set of numbers that are useful for something. It is possible and useful to confine your attention to what people might call the counting numbers or the Natural Numbers, so we do. That doesn't mean that mathematicians have never considered generalising the idea of prime numbers and investigating properties like prime factorisation in a structure bigger or more abstract than just the positive counting numbers - they certainly have.
There is already some terminology you could use to describe a set of things that behave like prime numbers but apply to a much more generalised set of objects than just the Natural numbers. These things are called "prime elements" and the parent algebraic structure is known as a "Ring". You seem to be interested in the Ring which is the Integers (positive and negative Naturals with 0, under conventional binary operations of + and x).
See Wikipedia entry: Prime elements, if you're interested. https://en.wikipedia.org/wiki/Prime_element
However, you should note that they exclude "units" which would include -1 in the ring of Integers, i.e. they would directly exclude both +1 or -1 from the prime elements in the ring of Integers. (For what reason? Similar to excluding 1 from the primes, it makes it much easier to state an equivalent unique factorisation theorem for the ring of Integers).
Here's a quick question or puzzle, just for fun. It relates to the idea you mentioned earlier of eliminating the number 2 from the prime numbers. You also seemed keen to extend beyond the positive numbers and consider negative numbers but you really don't have to stop there - you can consider Complex integers.
The Complex Integers or "Gaussian Integers" are the Complex number equivalents of integers. Specifically, the Gaussian integers are the set of all complex numbers of the form a+bi where a and b are integers.
Just like in ordinary arithmetic with Natural numbers, a prime (or prime element) of the Gaussian integers is a Gaussian integer, p, that is irreducible or cannot be factorised. Specifically, if we have p = q à r (where à is just ordinary multiplication of the complex integers q and r) then at least one of q or r must be a unit element. A "unit" is any complex number that lies on a unit circle around 0, so the only units in the Gaussian integers are +1, -1, +i, -i.
The number 2 is a prime in the ordinary integers. Is it still a prime in the Gaussian integers? To say that another way, can you factorise the number 2 in the Gaussian integers?
Spoiler: show
Best Wishes.
The following users thanked this post: chiralSPO
13
General Science / Re: Is 2 really prime? If so, why isn't 1?
« on: 28/04/2022 18:22:32 »
Hi. Fantastic diagrams. Great that you're making an effort to engage the audience with some Mathematics etc.
I wish you well.
I'll hide everything else under a spoiler because it's a bit dull and might prevent others from making their comments.
Best Wishes.
I wish you well.
I'll hide everything else under a spoiler because it's a bit dull and might prevent others from making their comments.
Spoiler: show
Best Wishes.
The following users thanked this post: chiralSPO
14
Physics, Astronomy & Cosmology / Re: Can sand/salt permanently molecules absorb resonate frequency?
« on: 22/02/2022 20:28:31 »The patterns formed on Chladni plates are a result of the properties of the plates themselves, and has nothing to do with the sand/salt/sugar/dust/etc. placed on top for visualization purposes. ..... The pattern that the nodes make is a function of the frequency of the vibration and the size/shape of the plate.Fully agree, acid test is that the pattern changes with frequency, so there is no memory effect.
PS I use tea leaves, but donât read anything into that

The following users thanked this post: chiralSPO
15
General Science / Re: co2 bomber extinguisher
« on: 16/01/2022 16:01:51 »Hi. I come up with extinguisher that can kill forest fires. I call it CO2 bomb.Fires need heat fuel and oxygen, you need to remove one to extinguish the fire. You cannot reliably remove the oxygen from a fire for a sustained period, nor the fuel in any reasonable scenario using fire suppressants etc, so you need to remove the heat. Extinguishing the fire may remove the heat from a small fire long enough to cool the fire but a large fire needs cooling down a great deal, this is why the fire brigade spend long periods dampening fires and raking through material piles.
Looks like American-football ball, but bigger. inside is lots of liquid CO2.
Drop one in the forest fire. Valves will open and release huge amount of liquid CO2, which will become gas.
Co2 from solid to gas I would bet has a far smaller energy requirement than liquid water to gas.
The following users thanked this post: chiralSPO
16
General Science / Re: Is this a feasible system for recycling CO2?
« on: 07/01/2022 16:55:47 »Alan, don't you work with MRIs? I assume you know what happens when a magnet quenches...Indeed. But suffocation incidents are usually associated with the loading and cooling process, not a subsequent quench. We have exhaust stacks to vent quench gas safely once the magnet is assembled.
My own MRI units used room-temperature resistive magnets or high-temperature supercons cooled with gaseous helium, but now I'm working with other people's kit, fraught with the dangers of liquid refrigerants.
Fortunately modern MRIs don't use nitrogen - one less problem - and capture helium boiloff, saving a lot of money. Time was that liquid helium was cheaper than beer when vast quantities were used for North Sea divers maintaining oil and gas rigs, but so much was exhausted to the cosmos that it is now more expensive than champagne.
The following users thanked this post: chiralSPO
17
Physics, Astronomy & Cosmology / Re: Energy loss in electrolysis
« on: 11/11/2021 08:41:49 »If you want light energy......Heat a piece of quicklime with your oxyhydrogen torch.
The following users thanked this post: chiralSPO
18
Technology / Re: What are some low-tech ways to address climate change?
« on: 08/11/2021 07:34:39 »A great way to reduce co2 or methane if you believe both to be the cause is to shoot animals, people will even pay you for the privilege.We fish and shoot already.
Animals arenât a problem because they release only âneutralâ carbon into the atmosphere. They release large amounts of CO2 and methane but their carbon source is limited to plants or animals that eat plants and plants get their carbon from the atmosphere. So, their only source of carbon is indirectly from the atmosphere and they only cycle carbon back to the atmosphere from whence it came. They do not cause a net gain in the amount of carbon. They recycle what is already there.
If it were not for animals, decomposition, insects, and fire would continue to recycle CO2 and methane back into the atmosphere in their place. If animals ate non-neutral carbon sources like coal or oil, that would be a problem because they would be releasing carbon from 300 million years ago back into the atmosphere and contribute to the net amount of atmospheric carbon. Fossil fuels are the problem.
The following users thanked this post: chiralSPO
19
General Science / Re: What's 0^0 ?
« on: 03/11/2021 00:53:20 »
Hi.
You're doing well there @hamdani yusuf .
You've already got enough to see that real numbers raised to some exponenet aren't always equal to a real number.
Sometimes, you can't find any real number that would be suitable. Sometimes you can find many suitable Real numbers. It's quite natural to extend the scope of the problem to consider complex numbers but then you can sometimes find an infinite set of numbers as a solution.
You already have enough evidence to recognise that 00 was never required to be a unique Real number. It's not even required to be a unique Complex number. You've also presented enough examples to see that it cannot be defined as a real number in any consistent way. If it has to be anything, it could be a bicycle. It's just a collection of mathematical symbols we can write down but it's not representative of any numerical value. ∀6â 12 is another set of symbols that doesn't equal or represent any numerical value.
Technically, x1/n is defined to be the positive root wherever there was a choice. So that 91/2 is +3 and nothing else. This is done because it maintains exponentiation as a well defined function for as long as possible. Anyway, using that it would mean that your answer to q. 4 is wrong.
If you were given the expression (x2)1/2 = x and then set x= -3 then you would have to deduce that +3 = -3.
Obviously we don't really want anything that silly, so the only possibile resolutions are that we give up on considering ab as a well defined function for all a,b ∈ Z OR ELSE accept that the given equation (x2)1/2 = x was not valid for all x∈ℜ.
Mathematics has taken the second option, the rules of manipulating indicies that we were taught in school do not hold for all real numbers as a base for the exponentiation. A good teacher might have brought that to the attention of their students but it wouldn't matter much anyway: As human beings we want to follow patterns and we want to extend these patterns wherever we can, so we would have ignored any warning.
Thus (xm)1/n = xm/n is only a true statement for some values of x. The original question 4 that I presented was a little misleading. The most appropriate response should be "Exponentiation cannot follow this rule even though it seems like it should (because otherwise +3 = -3)".
I needed this to be considered because there are so many misconceptions and false proofs based on using "rules of indicies" even though these rules do not and cannot hold in the system of mathematics we commonly use.
Best Wishes.
You're doing well there @hamdani yusuf .
You've already got enough to see that real numbers raised to some exponenet aren't always equal to a real number.
Sometimes, you can't find any real number that would be suitable. Sometimes you can find many suitable Real numbers. It's quite natural to extend the scope of the problem to consider complex numbers but then you can sometimes find an infinite set of numbers as a solution.
You already have enough evidence to recognise that 00 was never required to be a unique Real number. It's not even required to be a unique Complex number. You've also presented enough examples to see that it cannot be defined as a real number in any consistent way. If it has to be anything, it could be a bicycle. It's just a collection of mathematical symbols we can write down but it's not representative of any numerical value. ∀6â 12 is another set of symbols that doesn't equal or represent any numerical value.
Technically, x1/n is defined to be the positive root wherever there was a choice. So that 91/2 is +3 and nothing else. This is done because it maintains exponentiation as a well defined function for as long as possible. Anyway, using that it would mean that your answer to q. 4 is wrong.
If you were given the expression (x2)1/2 = x and then set x= -3 then you would have to deduce that +3 = -3.
Obviously we don't really want anything that silly, so the only possibile resolutions are that we give up on considering ab as a well defined function for all a,b ∈ Z OR ELSE accept that the given equation (x2)1/2 = x was not valid for all x∈ℜ.
Mathematics has taken the second option, the rules of manipulating indicies that we were taught in school do not hold for all real numbers as a base for the exponentiation. A good teacher might have brought that to the attention of their students but it wouldn't matter much anyway: As human beings we want to follow patterns and we want to extend these patterns wherever we can, so we would have ignored any warning.
Thus (xm)1/n = xm/n is only a true statement for some values of x. The original question 4 that I presented was a little misleading. The most appropriate response should be "Exponentiation cannot follow this rule even though it seems like it should (because otherwise +3 = -3)".
I needed this to be considered because there are so many misconceptions and false proofs based on using "rules of indicies" even though these rules do not and cannot hold in the system of mathematics we commonly use.
Best Wishes.
The following users thanked this post: chiralSPO
20
General Science / Re: What's 0^0 ?
« on: 02/11/2021 17:49:40 »
Hi.
The original question was:
It also turns out that whenever something like 00 is encountered in science, it has nearly always arisen as a Limt of xx as x→0+ (approaching 0 from the right). This limit is defined and does equal 1. As a consequence of this it has become an un-official convention that 00 = 1 and regrettably many calculators, like the one you were using, will show you that result.
Too often we start from a false assumption. It is easy to imagine that just because we can write some mathematical term down it must have some numerical value. For example, I have never calculated the value of 10067 + 765409 but I might assume it is some Real number. We also frequently assume that when there are patterns to follow we must be able to extend those patterns. For example, whatever my answer to that sum might be it should be bigger than the first number, 10067.
For exponentiation it's actually much safer if we start by assuming nothing at all. Do not assume ab defines any function from (a,b) → ℜ and don't even assume that the Real numbers exist. Instead start from more basic assumptions (axioms). If we do build up the Real Numbers and develop enough real Analysis to construct the exponential series then we will see that 00 was never defined and indeed it cannot be defined in any consistent way as a Real number.
I could just spit out some chapters from a textbook on Real Analysis or Complex Analysis that talk about the exponential series but I can't do that any better than the textbooks. Instead let's put out some minor problems to consider, which might help to identify just how complicated it is to raise numbers to an exponent:
1. What is 41/2 ? Why?
2. What is (-4)1/2 ? Is there no real solution?
4. By the rules of indicies we have (x2)1/2 = x for all x. This seems reasonable but what happens to the LHS and RHS when you let x = -3 ? Don't we obtain +3 = -3 ?
5. 3π cannot be written as the integer root of any integer power. Specifically 3π ≠ (a√3)b for any inetgers a,b. So what is the value of 3π? Could it be a negative number? If that's too easy consider (-3)π .
Best Wishes.
PS, yes I know question 3 was missing.
The original question was:
What's 0 to the power of 0?This is actually a very good question and it's something that isn't easily resolved.
It also turns out that whenever something like 00 is encountered in science, it has nearly always arisen as a Limt of xx as x→0+ (approaching 0 from the right). This limit is defined and does equal 1. As a consequence of this it has become an un-official convention that 00 = 1 and regrettably many calculators, like the one you were using, will show you that result.
Too often we start from a false assumption. It is easy to imagine that just because we can write some mathematical term down it must have some numerical value. For example, I have never calculated the value of 10067 + 765409 but I might assume it is some Real number. We also frequently assume that when there are patterns to follow we must be able to extend those patterns. For example, whatever my answer to that sum might be it should be bigger than the first number, 10067.
For exponentiation it's actually much safer if we start by assuming nothing at all. Do not assume ab defines any function from (a,b) → ℜ and don't even assume that the Real numbers exist. Instead start from more basic assumptions (axioms). If we do build up the Real Numbers and develop enough real Analysis to construct the exponential series then we will see that 00 was never defined and indeed it cannot be defined in any consistent way as a Real number.
I could just spit out some chapters from a textbook on Real Analysis or Complex Analysis that talk about the exponential series but I can't do that any better than the textbooks. Instead let's put out some minor problems to consider, which might help to identify just how complicated it is to raise numbers to an exponent:
1. What is 41/2 ? Why?
2. What is (-4)1/2 ? Is there no real solution?
4. By the rules of indicies we have (x2)1/2 = x for all x. This seems reasonable but what happens to the LHS and RHS when you let x = -3 ? Don't we obtain +3 = -3 ?
5. 3π cannot be written as the integer root of any integer power. Specifically 3π ≠ (a√3)b for any inetgers a,b. So what is the value of 3π? Could it be a negative number? If that's too easy consider (-3)π .
Best Wishes.
PS, yes I know question 3 was missing.
The following users thanked this post: chiralSPO
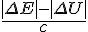
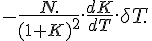
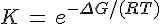 =
= 
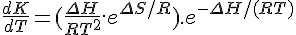
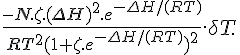
 Z
Z