What is Special Relativity?

Special relativity is a beautiful scientific theory; beautiful in the fact that it revolutionised the face of science by overthrowing Newtonian mechanics, and also beautiful in its simplicity. Einstein published his theory of special relativity in 1905; the theory describes how travelling at different speeds affects the length of objects and how fast or slow time passes. It is the simplest form of general relativity, as it only considers objects travelling at a constant speed - hence the title 'special'. Accelerating objects are left for general relativity to explain. Einstein published his complete theory of general relativity in 1915, ten years after special relativity; this year marks the 100th anniversary of his publication.

Although special relativity is little heard of, it plays an integral part in all our lives. Alterations in length and time due to special relativity have to be accounted for in everyday objects, such as global positioning systems (GPSs). Because of the genius persona that society has assigned Einstein, it is often falsely assumed that special relativity is too hard to understand, when in fact the whole of special relativity is actually derived from two very simple principles.

The Principles of Special Relativity

The First Principle of Special Relativity

You are sitting on board a train, willing the train to pull away. Finally you hear the beeping doors, indicating your train is about to depart. You look out of your window at the train on the adjacent track and watch as you glide past it. Only, once you've pulled past the other train you don't see the world rushing past, but the completely stationary station. It was the other train that pulled off in the opposite direction.

Everyone can relate to this story, it has happened to all of us. This is because, once you have stopped accelerating, there is no way to tell if your train is moving or not. Once you are moving, everything inside the train behaves as though you are stationary; your cup stays still on your table and you can walk up and down your carriage freely, as if it's not hurtling forward at 60 mph. This is because the laws of physics are the same regardless of whether you are stationary or moving at a constant speed.

But, when you look out of the window and see the world zooming past, does that not tell you, you are moving? No, it does not! All that tells us is that we are moving relative to the Earth, but we can't really know how fast we are moving, or even if we are moving at all, as the Earth itself isn't stationary. The Earth is orbiting the sun, the sun is orbiting the center of our galaxy, and our galaxy is also constantly rotating, not to mention that the universe is constantly expanding.

There is no way to know if something is moving or not; this is the first principle of special relativity.

The Second Principle of Special Relativity

You and your friend Erwin each buy a high-speed rocket to fly in, and decide to show off to your friend Rosalind. You have a race; Rosalind stands on earth looking up at you and measures your speeds. She measures your speed to be 20,000mph and Erwin's to be 15,000mph.

However, Erwin is also measuring your speed and in his opinion you are travelling at only 5,000mph. That is because 5,000mph is the difference between your two speeds. Therefore, you have no absolute speed, how fast you are travelling depends on how fast the observer is travelling in comparison to you.

To test this observation further, you launch a ball out of the front of your rocket at 1,000mph. To you the ball is travelling at 1,000mph, Rosalind measures it to be travelling at 21,000mph and Erwin measures it to be travelling at 6,000mph. This again demonstrates how speed is relative, the ball has no absolute speed of its own and its speed depends on how fast the observer is travelling in comparison to it.

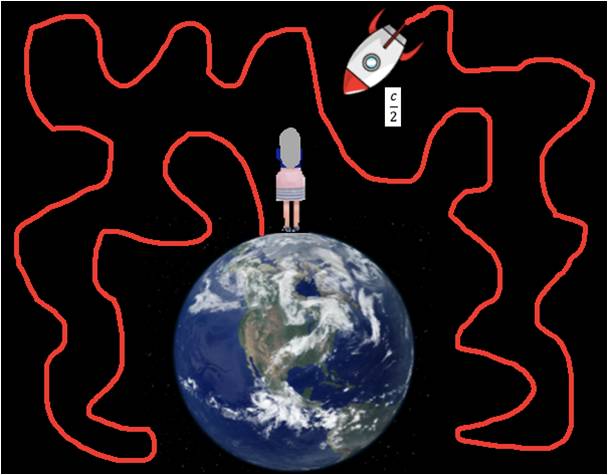
Both you and Erwin discover a new button which turns on super powerful thrust engines in your rockets. When you press your button you travel at half of the speed of light (c/2), and when Erwin presses his button he travels at one quarter of the speed of light (c/4). You decide to test to see if your previous observations also apply to light; you flash your torch, releasing a beam of light. You measure the speed of the beam to be the speed of light (c). Based on your previous observations, you would expect Rosalind to measure the speed of the beam to be 1.5c, because she observes a beam ejected at speed c, from a rocket travelling at c/2. However, this is not what happens, Rosalind also measures the beam to be travelling at c. In fact, Erwin also measures your beam to be traveling at c. You, Rosalind and Erwin all record the beam to be travelling at exactly the same speed.

The speed of light is the same for all observers; this is the second principle of special relativity.

How can the speed of light be the same for all observers?

If you think that this is extremely strange, you are not alone. The fact that light travels at the same speed for all observers directly contradicts the previous example using the ball. However, it can be explained. We all know that:

Speed = distance/time

In order for all observers to agree on the speed of light, distance and time change depending on the observer's speed. The faster you are travelling the slower time moves and the more objects shrink! So your watch is moving slower than Erwin's watch and Erwin's watch is moving slower than Rosalind's watch; a ruler on board your rocket is shorter than a ruler on board Erwin's rocket and Erwin's ruler is shorter than Rosalind's. These alterations mean that everyone measures the speed of light to be the same.

For speeds that aren't near the speed of light, time and distance change by a minuscule amount. Every time you get the train, time does slow down and objects do shrink, but by such a small amount that you could never detect it. However, there are instances this difference is significant.

Special Relativity in Action

If we were able to travel closer to the speed of light, special relativity would have large consequence. For example, you decide to go off in your rocket and travel round space at half the speed of light (c/2), for four years. However, when you get back you find that in fact 30 years have past for Rosalind! This is because you were traveling faster than the earth so time was passing slower for you than for Rosalind - resulting in you only aging four years compared to her 30.

Although examples like the one above do not yet occur in everyday life, the effects of special relativity do frequently have to be accounted for, especially in electronics such as GPSs. Most people have probably used a GPS. They are so commonplace that it is easy to forget how clever they really are; you can stand anywhere in the world with a device as small as your phone, and be told your position on the globe to within less than half a meter!

GPS receivers work by receiving a signal from at least four out of 24 of the positioning satellites we currently have in orbit around the earth. Each emits a signal, which is picked up by the receiver. The receiver then calculates its position by measuring the time taken for each signal to arrive from the satellites, which it knows the positions of. To ensure that the receiver and the satellite agree on the time the signal is sent, special relativistic effects have to be accounted for. Due to the fact that an observer on the ground sees the satellites moving relative to them, special relativity predicts that their clocks should move more slowly. If this difference in time were not accounted for, errors in position would occur at a rate of ten kilometers per day!

2015 marks the 100th anniversary of one of the most ground breaking scientific papers ever produced; a paper that gave the world space travel, satellites, GPS and a greater understanding of our universe. Let's hope we don't have to wait another 100 years for the next one.